

Piezo Accelerometer Tutorial
What is Vibration?
What is Vibration?
Before we look into accelerometers we want to get a basic understanding of the physics of vibration.
In some places it will be necessary to dive into the theory (sorry for that) but if you don't understand every detail you still can continue.
If you don't have a technical background, please follow the green path, as it takes a logical approach to all topics and touches on the basics for understanding.
In certain instances, a yellow path will take you to a deeper level. If the green path is too simple for you, the yellow path and the red pages may be more suitable.
The additional mathematical information is provided on the red pages.
Physics of Vibration
Acceleration
Acceleration has something to do with movement.
The physical movement of a body can be described with the velocity v of that body.
The velocity has a magnitude (i.e. how fast the body is moving) and a direction (i.e. where the body is moving to).
Such a physical quantity is called a vector and is represented by an arrow whose length corresponds to the magnitude and is pointing in the direction.
If the velocity (in magnitude and direction) is constant the body is moving steadily. We say it is in uniform movement. However the velocity can also change with time.
The change in velocity Δv of a body (either in it’s magnitude or in the direction) during the time period Δt is called acceleration a.
a = Δv / Δt
Δv = v₂ -v₁ Δt = t₂ -t₁
The Greek letter Δ (Delta) is used in maths to indicate a difference between to values. Here namely the difference between the velocity v₁ at the time t₁ and v₂ at t₂.
The velocity is measured in meters per second m/s.
The dimension of the acceleration (change in velocity per second) becomes therefore meters per second per second
m/s/s or m/s²
The acceleration is also a vector.
I.e. it has a magnitude and a direction.
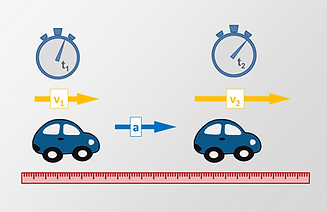
At time t₁ the velocity of the car is v₁, at the time t₂ it is v₂. The acceleration a is the change in velocity between t₂ and t₁
The acceleration is the difference of the velocities at t₂ and t₁ divided by the time difference t₂ - t₁
If you like more maths, try out the
Sir Isaac Newton
A particular acceleration that we feel every day is the gravity of the earth which makes things fall down on the ground when they are let go.
Sir Isaac Newton, an English physicist and mathematician formulated the laws of motion and universal gravitation in 1687.
Newton himself often told the story that he was inspired to formulate his theory of gravitation by watching the fall of an apple from a tree.
The gravitational acceleration is 1g = 9.81 m/s²

Isaac Newton © by Wikipedia, the free encyclopedia
Other ground-breaking apple stories:
Uniform Acceleration
also linear acceleration, particularly gravitation
A body under uniform acceleration a changes its velocity v steadily during the time t :
v = a · t
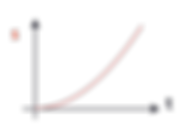
The traveled distance s is increasing quadraticly with time.
s = a / 2 · t ²
A particular case is the free fall of a body.
In this case the acceleration is
1 g = 9,81 m/s² (g like gravitation).


Newton's Law
When a body with the mass m is accelerated with an acceleration a then the force F is acting on the body.
F = m · a
The dimension of a force is called Newton [N].

1 Newton [N] is the force that accelerates 1 kg
mass with 1m/s²
or
If a body with1 kg mass is accelerated with 1m/s²
then a force of 1 Newton [N] is acting on that body.
1 N equals about to the weight of a regular chocolate bar of 100gr.

Alternating Acceleration
The motion of a mass attached to a spring presents a particular case where the acceleration changes its magnitude and direction periodically.
When the mass is lifted and left to itself it oscillates on the resonance frequency of the system.



